紙にインク, 210mm × 297mm, 2002年 ／ Ink on Paper, 210mm × 297mm, 2002.

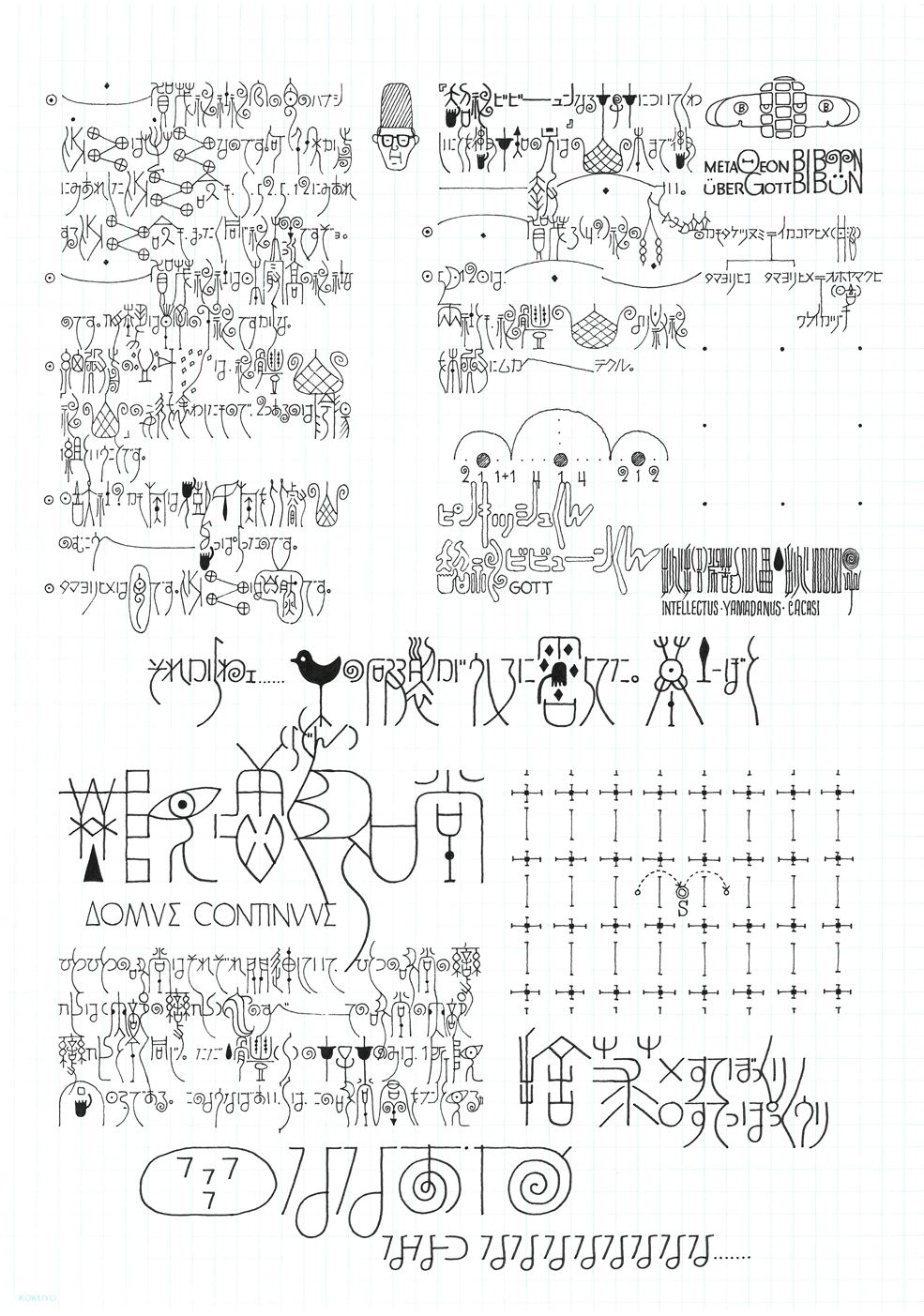
ひとつひとつの聖堂はそれぞれ関連していて、ひとつの聖堂の変化は（内部の変化）他のすべての聖堂の内部変化と全く同じ。ただ主体（S）の存在のみは１所限定的である。このようなばあい、Sはこの聖堂群をなんと見るか

この問題は、ライプニッツの『モナドロジー』で論じられている問題と全く同じであると後に気がついた。すべてのモナドの内部の変化が他のモナドとまったく同じであれば、それぞれのモナドは相互に区別できなくなって、結局宇宙には、ただ一つのモナドしか存在しなくなってしまう。しかし、現実の宇宙がそうでないかぎり、モナドの内部はすべて異なっている。「白紙」は、すべての部分がみな同じに見えるけれど、それが存在であるかぎり、すべての部分はみなそれぞれに異なっている。白紙に点が打たれると、それはマトリックスとなって存在の表から姿を消す。

Each dome is related to others, and the internal change of one is identical to the internal change of all others. Only the existence of the subject(S) is only one place-limited. In this case, what does S consider this domes group to be?

Later, I noticed that this problem was identical to a problem discussed in "monadology" of Leibniz. If the change in all monads is identical to other monads, you cannot distinguish each monad mutually, and only one monad merely exists in the universe after all. However, all the inside of the monad is different unless real universe is so. "The blank paper" varies in all parts as far as it is existence though all parts look same. When a point is pictured to a blank paper, it becomes the matrix and disappears from the surface of the existence.